The negative binomial distribution
[this page | pdf | back links]
The negative binomial distribution describes the probability
of 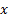 successes in a
sequence of independent experiments each with likelihood of success of
successes in a
sequence of independent experiments each with likelihood of success of 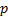 that
arise before there are
that
arise before there are 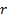 failures. In this
interpretation
failures. In this
interpretation 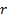 is a positive
integer, but the distributional definition can also be extended to real values
of
is a positive
integer, but the distributional definition can also be extended to real values
of 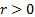 . Note: different
texts adopt slightly different definitions, e.g. with support starting at
. Note: different
texts adopt slightly different definitions, e.g. with support starting at 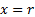 not
not
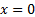 and/or with
and/or with 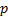 denoting
probability of failure rather than probability of success.
denoting
probability of failure rather than probability of success.
|
Distribution name
|
Negative
binomial distribution
|
|
Common notation
|
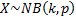
|
|
Parameters
|
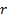 =
number of failures ( =
number of failures (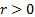 ) )
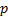 =
probability of success in each experiment ( =
probability of success in each experiment (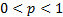 ) )
|
|
Support
|
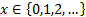
|
|
Probability mass
function
|
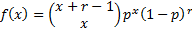
If 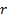 is non-integral
then is: is non-integral
then is:
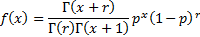
|
|
Cumulative distribution
function
|
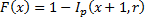
|
|
Mean
|

|
|
Variance
|
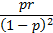
|
|
Skewness
|

|
|
(Excess) kurtosis
|
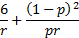
|
|
Characteristic function
|
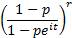
|
|
Other comments
|
The geometric distribution is the same as the
negative binomial distribution with parameter 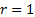 .
Its pdf and cdf are therefore: .
Its pdf and cdf are therefore:
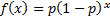
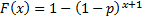
For the special case where 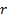 is
an integer the negative binomial distribution is also called the Pascal
distribution. The Poisson
distribution is also a limiting case of the negative binomial: is
an integer the negative binomial distribution is also called the Pascal
distribution. The Poisson
distribution is also a limiting case of the negative binomial:
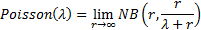
|
Nematrian web functions
Functions relating to the above distribution may be accessed
via the Nematrian
web function library by using a DistributionName of “negative
binomial”. For details of other supported probability distributions see here.
NAVIGATION LINKS
Contents | Prev | Next