Enterprise Risk Management Formula Book
Appendix A.5: Probability Distributions: Multivariate
probability distributions
[this page | pdf | back links]
Multivariate normal (i.e. Gaussian) distribution
The multivariate probability distribution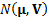 
where 
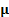is a vector
of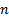 
elements
and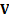 
is an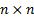 
non-negative
definite matrix has the following joint density function (where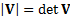 
is the
determinant of V)
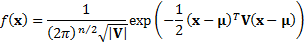
The means of the individual marginal distributions are 
where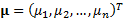 
and the
covariance between the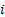 
’th and the 
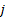’th
marginal distributions are 
where the are the
elements of 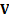. Its moment
generating function is 
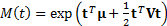and its
characteristic function is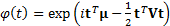 
. The
multivariate normal distribution has as its copula the Gaussian copula.
A bivariate random variable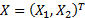 
follows a standard
bivariate normal distribution if it has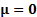 
and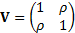 
. More
generally, a multivariate normal distribution is a standard multivariate normal
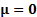distribution if and
a covariance matrix which is also a correlation matrix, i.e. where the variance
of each individual marginal distribution is 1.
For numerical values of the cumulative distribution function
of the standard bivariate normal distribution see here.
NAVIGATION LINKS
Contents | Prev | Next