Continued Fractions

[this page | pdf | references | back links]

A continued fraction (characterising a function in one variable) has the following form:

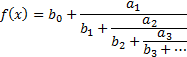
Publishers tend to prefer to write this as:

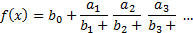
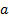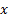In either format the ’s and ’s can themselves be functions of  (usually linear or quadratic at worst, i.e. low order polynomials in ).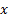

Continued fractions are often powerful ways of numerical evaluation of functions since they often converge much more rapidly than power series expansions. According to Press et al. (2007) they often also have a larger domain of convergence in the complex plane, which means that they can prove an effective way of evaluating a function for a wider range of input values than might be practical with a power series expansion.