Calibrating probability distributions
used for risk measurement purposes to market-implied data: 2. Multi-instrument
calibration – Section Analysis
[this page | pdf | references | back links]
Return
to Abstract and Contents
Next
Page
2.3 A multivariate normal distribution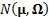 
with
mean 
(a
vector of random variables) and covariance matrix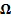 
has a
probability density function,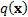 
as
follows, where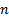 
is the
number of entries in the vector and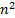 
the
number of entries in ):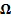
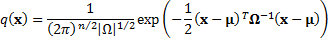
2.4 We note that:
(a) Any 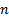-dimensional
multivariate normal distribution has a probability density function expressible
as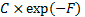 
where 
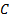is some
suitable constant and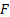 
is a
positive definite symmetric quadratic form (with possibly non-zero drift) in 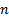different
variables, and vice versa.
(b) Applying analytical
weighted Monte Carlo (using relative entropy) to the sort of calibration
problem referred to above will therefore return (unless the calibration problem
is ill-posed) a calibrated probability distribution which also has multivariate
normal form. This is because the problem can be restated using Lagrange multipliers
to one that involves minimising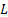 
defined
as follows, where the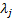 
refer
to whatever calibrations there are on the means and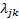 
to
those on covariance terms (in general there will be fewer than 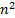of the ):
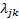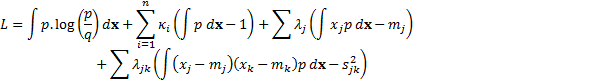
The solution to this minimisation
problem is given by the following:
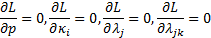
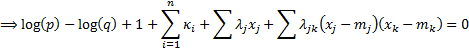
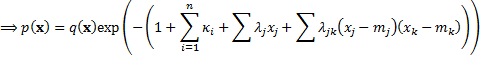
subject to 
(i.e.
that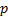 
is a
probability distribution) and other constraints derived directly from
calibration requirements, e.g. that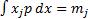 
etc.
Thus if 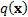is
expressible as 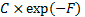as
above, then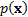 
will be
too, just for a different .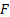
(c) Applying the
principle of no arbitrage we may therefore expect 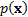to have
zero mean (more precisely for each element of to be
the same, which without loss of generality we may take as zero if we are
focusing on relative returns) and therefore to have the form:
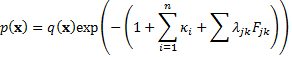
where the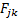 
are
symmetric zero-drift quadratic forms (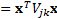
, say)
corresponding to each of the implied volatilities/implied correlations to which
we wish to calibrate.
(d) The calibrated
distribution will therefore be multivariate normal with zero mean and
probability distribution as follows, for suitably chosen 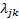that
reproduce for the calibrations the relevant market implied variances or
covariances (where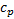 
is some
constant the value of which ensures that ):
(e) Thus the calibrated
probability distribution will be characterised by a covariance matrix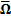 
as
follows:
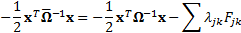
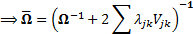
NAVIGATION LINKS
Contents | Prev | Next